Projects
On this page, I give an overview over research projects that I currently work on or that I have been working on in the past. If you are interested in learning more about these projects, do not hesitate to contact me.
String Geometry and Functorial Field Theory

About this project
Motivated by Witten's work on the index of the Dirac operator on loop space, the goal of this project is to understand the spin geometry of the loop space of Riemannian manifolds. Differential geometric objects on the loop space often correspond to higher-categorical geometric objects on the manifold itself. String geometry studies the interplay between these two points of view.
Talks given about this project
| Video |
The spinor bundle on loop space and its fusion product
Center for Quantum and Topological Systems at NYU Abu Dhabi |
|
| Video |
The stringor bundle of a string manifold
Mid-South Algebraic Topology and Geometry Workshop |
|
| Video |
The stringor bundle (by Konrad Waldorf)
"QFT and Cobordism" @ Center for Quantum and Topological Systems at NYU Abu Dhabi |
|
| Video |
The stringor bundle (by Konrad Waldorf)
Galileo Galilei Institute |
|
| Video |
Approaching string geometry through loop spaces (by Peter Kristel)
Mid-South Algebraic Topology and Geometry Workshop |
Publications relevant to this project
| arXiv | A representation of the string 2-group, with Peter Kristel and Konrad Waldorf. arXiv preprint |
||
| arXiv | The spinor bundle on loop space. arXiv preprint |
||
| arXiv | The stringor bundle, with Peter Kristel and Konrad Waldorf. arXiv preprint |
||
| arXiv | The insidious bicategory of algebra bundles, with Peter Kristel and Konrad Waldorf. arXiv preprint |
||
| arXiv | Journal | Lie 2-groups from loop group extensions, with Konrad Waldorf. J. of Homotopy and Rel. Structures 19, pp 597-633, 2024 |
|
| arXiv | Journal | Categories of Lagrangian Correspondences in Super Hilbert Spaces and Fermionic Functorial Field Theory. SIGMA 20, paper no. 036, 2024 |
|
| arXiv | Journal | The Clifford algebra bundle on loop space. SIGMA 20, paper no. 020, 2024 |
|
| arXiv | 2-vector bundles, with Peter Kristel and Konrad Waldorf. Accepted for publication in Higher Structures |
||
| arXiv | Journal | A framework for geometric field theories and their classification in dimension one, with Augusto Stoffel. SIGMA 17, paper no. 072, 2021 |
|
| arXiv | Journal | The Chiral Anomaly of the Free Fermion in Functorial Field Theory, with Saskia Roos. Ann. Henry Poincaré 21, pp 1191-1233, 2020 |
Mathematics of Topological Insulators
About this project
Topological insulators are certain physical materials or metamaterials that are insulating in the interior, but have remarkable conductivity properties at their boundary. Because of this unusual feature, topological insulators are a highly active topic in condensed matter physics. Here the term "topological", comes from the fact that these materials have a certain topological non-trivialities in their mathematical description, which can be described by K-theoretic or field theoretic invariants. In this project, we study the mathematics underlying these physical systems using the theory of coarse geometry developed by Roe and others.
Talks given about this project
| Video |
Spectral aspects of topological matter with an eye on strings (by Guo Chuan Thiang)
Au & NZ Geometry Strings Fields Seminar |
|
| Video |
What is a Coarse Index, physically? (by Guo Chuan Thiang)
Global Noncommutative Geometry Seminar, |
Publications relevant to this project
| arXiv | Coronas and Callias type operators in coarse geometry, with Ulrich Bunke. arXiv preprint |
||
| arXiv | Quantization of conductance and the coarse cohomology of partitions, with Guo Chuan Thiang. arXiv preprint |
||
| arXiv | Journal | Coarse geometric approach to topological phases: Invariants from real-space representations, with Christoph Setescak and Caio Lewenkopf. Phys. Rev. B 111, paper no. 104207, 2025 |
|
| arXiv | Book | Coarse geometry and its applications in solid state physics. Encyclopedia of Mathematical Physics, 2nd Edition, Editors: Emile Prodan, Elsevier |
|
| arXiv | Journal | Large-scale geometry obstructs localization, with Guo Chuan Thiang. J. Math. Phys. 63, paper no. 91902, 2022 |
|
| arXiv | Journal | Delocalized spectra of Landau operators on helical surfaces, with Yosuke Kubota and Guo Chuan Thiang. Comm. Math. Phys. 395, pp 1211-1242, 2022 |
|
| arXiv | Journal | Breaking symmetries for equivariant coarse homology theories, with Ulrich Bunke. J. Geom. Phys. 201, paper no. 105214, 2024 |
|
| arXiv | Journal | Gaplessness of Landau Hamiltonians on hyperbolic half-planes via coarse geometry, with Guo Chuan Thiang. Comm. Math. Phys. 386, pp 87-106, 2021 |
|
| arXiv | Journal | Cobordism invariance of topological edge-following states, with Guo Chuan Thiang. Adv. Theor. Math. Phys. 26(3), pp 673-710, 2022 |
|
| arXiv | Journal | Good Wannier bases in Hilbert modules associated to topological insulators, with Guo Chuan Thiang. J. Math. Phys. 61, paper no. 061902, 2020 |
Path Integrals in Geometry and Physics
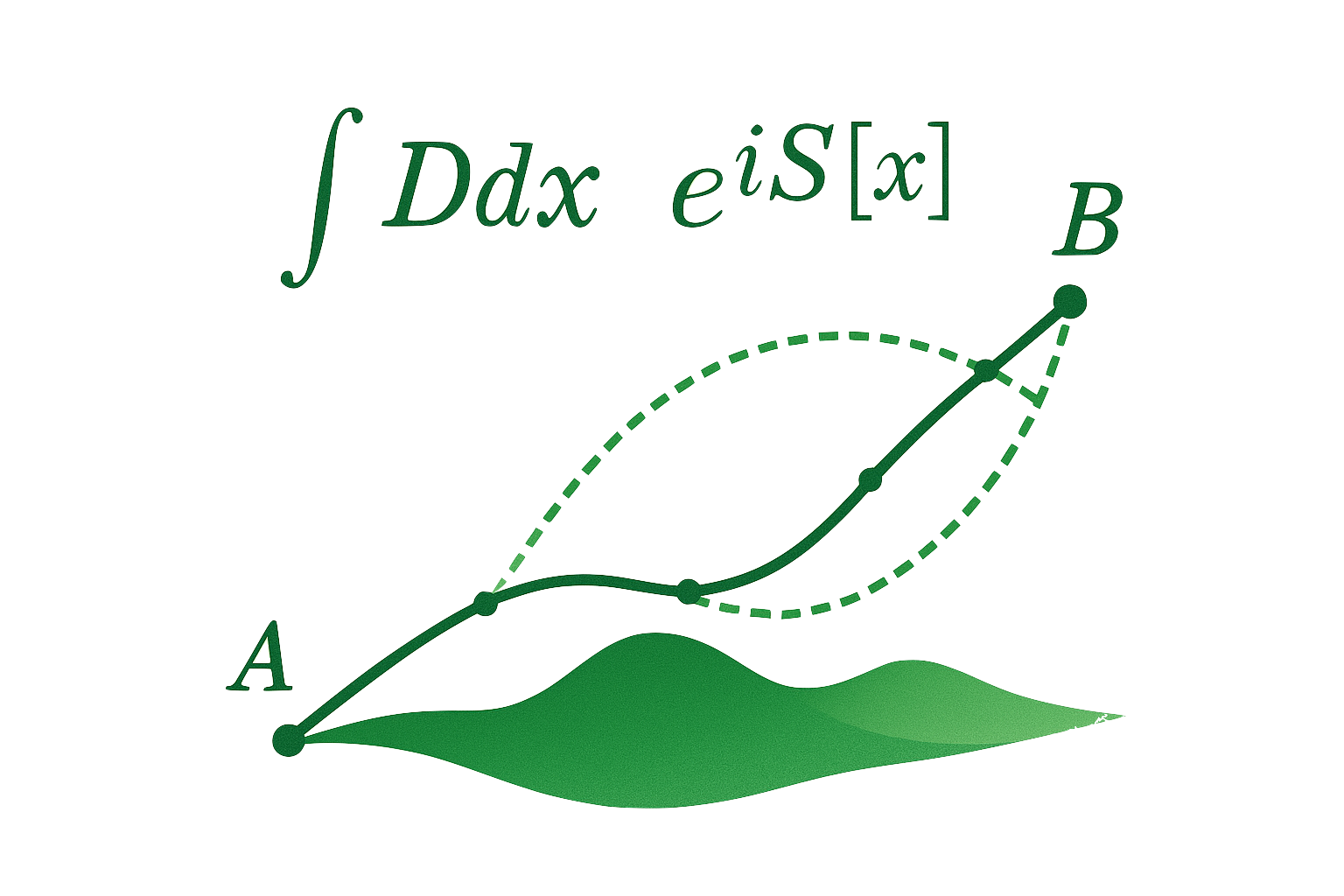
Publications relevant to this project
| arXiv | Journal | A Short Proof of the Localization Formula for the Loop Space Chern Character of Spin Manifolds, with Zelin Yi. J. Noncommut. Geom. 17(1), pp 189-210, 2023 |
|
| arXiv | Book | Construction of the Supersymmetric Path Integral: A Survey. Differential Geometry in the Large, Editors: Owen Dearricott, Wilderich Tuschmann, Yuri Nikolayevsky, Thomas Leistner, Diarmuid Crowley, Cambridge University Press |
|
| arXiv | Journal | The Chern Character of theta-summable Fredholm Modules over dg Algebras and the Supersymmetric Path Integral, with Batu Güneysu. Adv. Math. 395, paper no. 108143, 2021 |
|
| arXiv | Book | Heat Kernels as Path Integrals. Space – Time – Matter, Editors: Jochen Brüning and Matthias Staudacher, de Gruyther |
|
| arXiv | Journal | The Fermionic integral on loop space and the Pfaffian line bundle, with Florian Hanisch. J. Math. Phys 63, paper no. 123502, 2022 |
|
| arXiv | Journal | A Rigorous Construction of the Supersymmetric Path Integral Associated to a Compact Spin Manifold, with Florian Hanisch. Comm. Math. Phys 391, pp 1209-1239, 2022 |
|
| arXiv | Journal | Heat kernel asymptotics, path integrals and infinite-dimensional determinants. J. Geom. Phys. 131, pp 66-88, 2018 |
|
| arXiv | Journal | Strong short-time asymptotics and convolution approximation of the heat kernel. Ann. Global Anal. Geom. 55, pp 371-394, 2019 |
|
| arXiv | Journal | Path Integrals on Manifolds with Boundary. Comm. in Math. Phys. 354, pp 621-640, 2017 |
Heat Kernels and Geometric Invariants

Publications relevant to this project
| arXiv | Journal | The Trace and the Mass of subcritical GJMS Operators. Diff. Geom. Appl. 56, pp 95-109, 2018 |
|
| arXiv | Journal | Asymptotic Expansions and Conformal Covariance of the Mass of Conformal Differential Operators. Ann. Global Anal. and Geom. 52, pp 237-268, 2017 |
|
| arXiv | Journal | Strong short-time asymptotics and convolution approximation of the heat kernel. Ann. Global Anal. Geom. 55, pp 371-394, 2019 |